Insider Brief:
- Silicon Quantum Computing (SQC) executed Grover’s algorithm on a four-qubit silicon processor with 93.46% accuracy, achieving 98.87% of the ideal theoretical probability (94.53%) without error correction.
- SQC’s silicon qubits, made from phosphorus atoms in isotopically purified silicon, demonstrated single-qubit fidelities above 99.9% and two-qubit fidelities over 99%. These fidelities exceeded the fault-tolerance threshold, allowing Grover’s algorithm to run successfully without requiring error correction.
- Despite this success, the demonstration remains a small-scale proof of concept. The experiment used only three nuclear spin qubits within the four-qubit processor, far from the scale needed to achieve quantum advantage over classical computing.
- SQC’s results suggest that high-fidelity qubits could reduce reliance on extensive error correction, but scalability remains a critical challenge. Maintaining these fidelity levels as the system expands will determine whether this approach can compete with error-corrected quantum architectures.
In searching a vast, unstructured database, one with billions of entries, no shortcuts, and no indexes, a classical computer would check each entry one by one, a process that could take an impractical amount of time. Grover’s algorithm, one of the foundational algorithms in quantum computing, provides a fundamentally different approach, with the potential to search such a database quadratically faster than classical methods. While the promise of quantum search is compelling, its realization depends on the hardware that runs it.
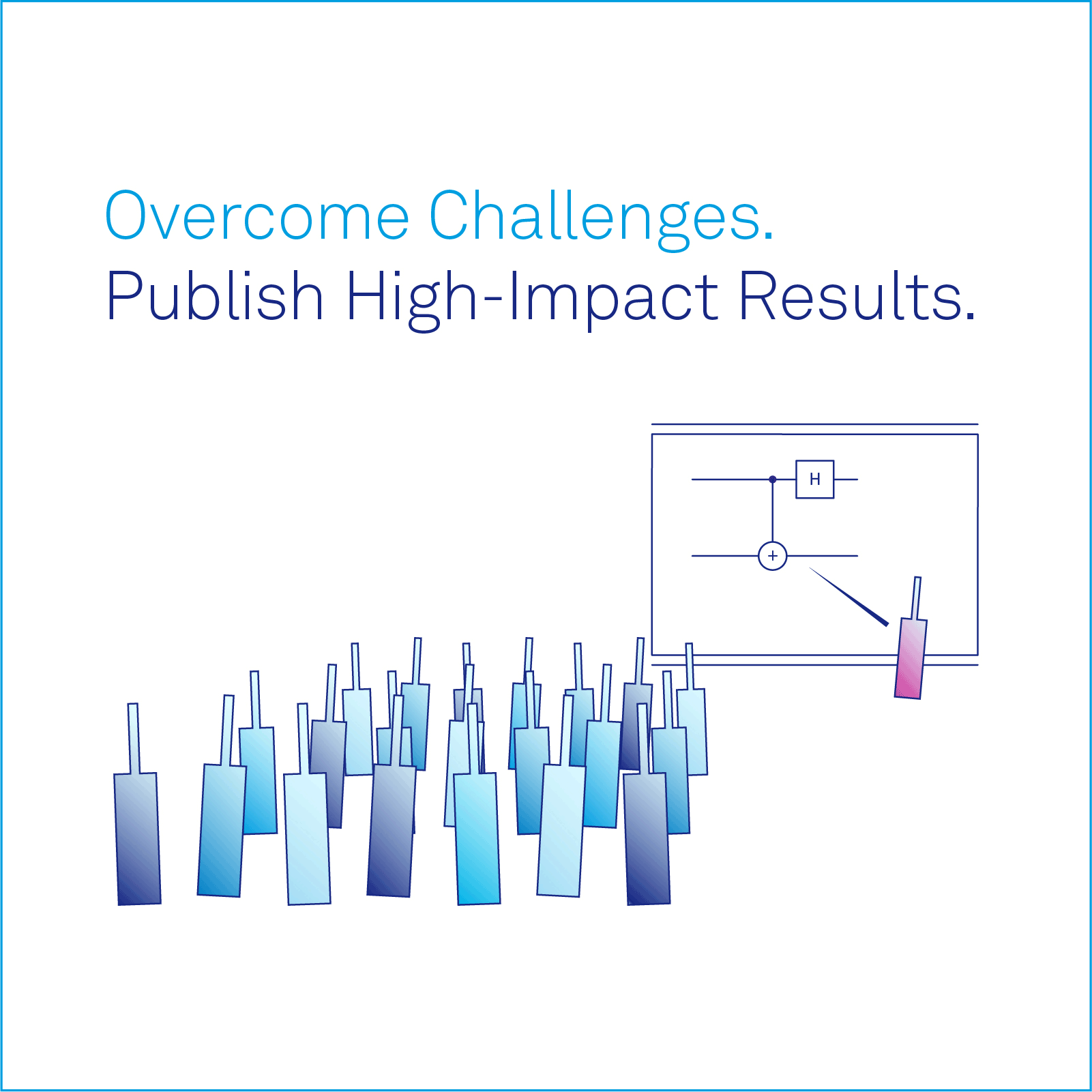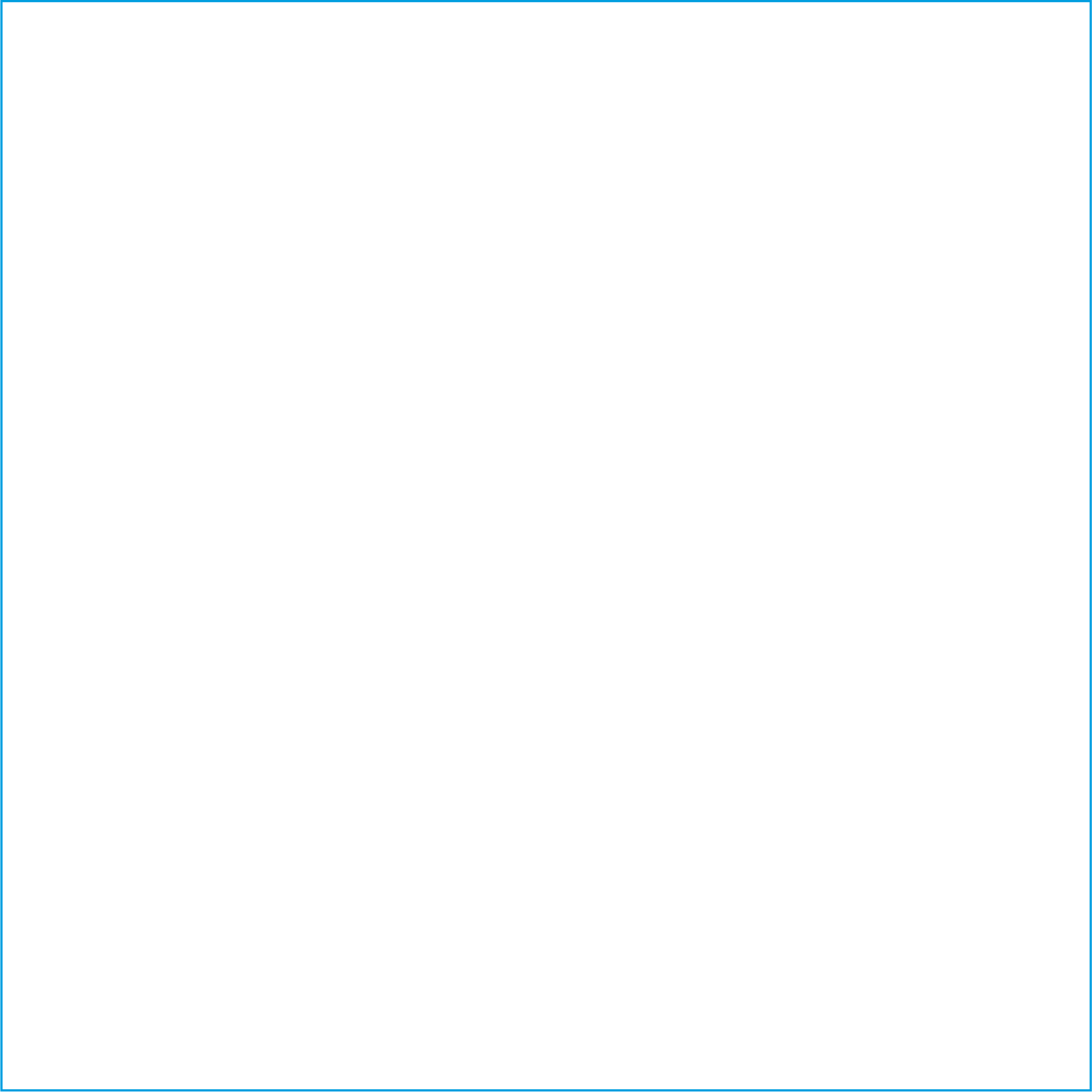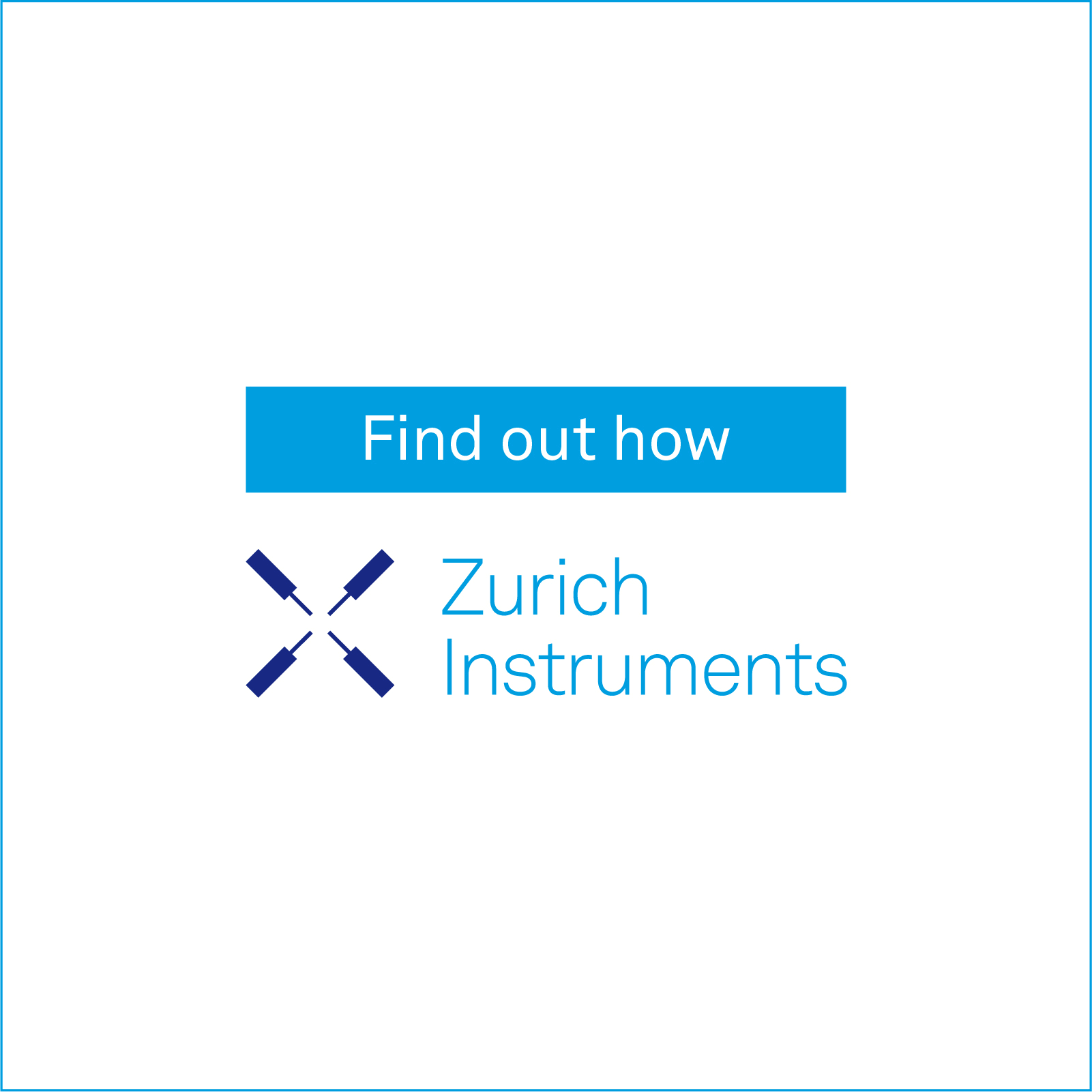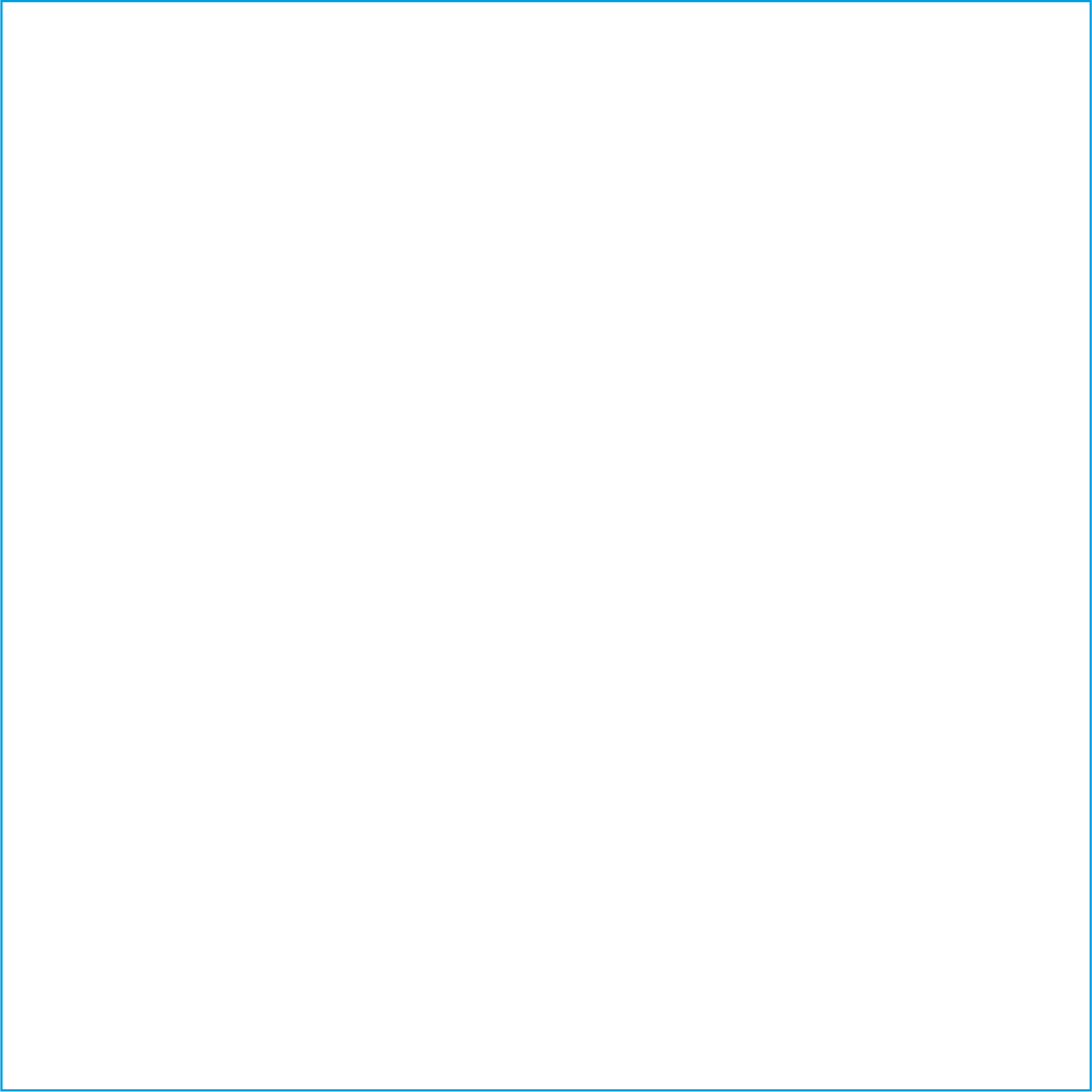
Silicon Quantum Computing has reported a high-accuracy implementation of Grover’s algorithm on a four-qubit silicon processor, achieving a 93.46% (98.87% of the ideal probability 94.53%) probability of correctly identifying the marked state. The study, published in Nature Nanotechnology, demonstrates results that speak to the advantages of high-fidelity qubits and atomic precision manufacturing in advancing quantum computing. Importantly, this was achieved without error correction, which exemplifies the potential of SQC’s hardware. While the demonstration represents an improvement in error rates, it also reinforces the ongoing challenges of scaling beyond small, proof-of-concept systems.
Grover’s Algorithm as a Fundamental Benchmark, Not Yet a Breakthrough
Grover’s algorithm, first introduced in 1996, is one of the fundamental quantum algorithms that provides a theoretical quadratic speedup for searching an unsorted database. While the algorithm is often cited as evidence of quantum computing’s potential, its real-world applicability depends on the quality of the hardware executing it.

SQC’s demonstration used a four-qubit silicon-based system, which is small compared to the scale needed for practical quantum computing. Grover’s algorithm theoretically achieves near-perfect results in an ideal quantum system, but in reality, quantum processors are limited by error rates and decoherence. The key result in SQC’s work is not simply the execution of Grover’s search—which has been implemented on other platforms—but the fact that it was done with high fidelity exceeding the fault-tolerance threshold, without requiring error correction.
According to a recent release, the team achieved up to 98.9% accuracy of the theoretical maximum for Grover’s algorithm. However, it’s important to recognize that four qubits remain far from the scale needed for quantum advantage, and running Grover’s algorithm on such a small system does not yet translate into a computational advantage over classical methods.
Clarification of Grover’s Algorithm Accuracy
SQC’s implementation of Grover’s algorithm identified the correct state with a 93.46% success rate, compared to the ideal theoretical success probability of 94.53% in a perfect quantum system. This means that SQC’s execution reached 98.87% of the ideal probability, a strong indicator of the processor’s high-fidelity operation.
To break this down: in an ideal noise-free quantum system, Grover’s algorithm with two iterations (r = 2) should output the correct result 94.53% of the time. However, real quantum hardware is susceptible to errors and decoherence, which typically reduce success rates. SQC’s 93.46% result shows that their hardware performed very close to the theoretical limit, achieving 98.87% of the ideal probability.
While this level of accuracy, especially without error correction, highlights the quality of SQC’s qubits, it is important to recognize that Grover’s algorithm was run on only three nuclear spin qubits within the four-qubit processor, meaning this result remains a small-scale proof of concept rather than a demonstration of large-scale quantum advantage.
The Real Story is in High-Fidelity Qubits
Where SQC’s work is intended to stand out is not in the number of qubits but in how well they perform. Unlike many quantum computing efforts that prioritize increasing qubit count, SQC has focused on improving qubit stability, coherence times, and gate fidelities.
Their silicon qubits are phosphorus atoms embedded in isotopically purified silicon, avoiding the variability introduced by other methods of qubit fabrication. This enables single-qubit gate fidelities exceeding 99.9% and two-qubit gate fidelities above 99%, surpassing the threshold required for fault-tolerant quantum computation. These fidelities allowed SQC to run Grover’s algorithm successfully without relying on error correction, which is typically needed to mitigate hardware limitations in other quantum platforms.
In the release, Michelle Simmons, Founder and CEO of SQC, emphasized the importance of quality over quantity stating “In the race to deliver commercially viable quantum computers, what ultimately matters is not how many qubits you have, but the quality of your qubits. Throwing thousands or millions of low-quality qubits at the problem is not going to work.”
This philosophy is reflected in SQC’s atomic-scale fabrication approach, which precisely places qubits at the atomic level using scanning tunneling microscopy (STM) lithography. Unlike competitors who rely on third-party chip manufacturers, SQC states that their in-house process allows them to iterate designs in as little as one to two weeks, which accelerates hardware improvements.
A Step Forward, But Challenges Remain
While SQC’s results are promising, they also reveal the challenges ahead. A four-qubit system, even with high fidelity, is not yet capable of solving problems beyond classical reach. Fault-tolerant quantum computing will require hundreds or thousands of logical qubits, which means scaling remains a major hurdle.
Additionally, while silicon spin qubits offer long coherence times and compatibility with existing semiconductor manufacturing, scaling up while maintaining atomic precision is an unsolved challenge. Many large-scale quantum computing efforts rely on error correction techniques that require a high number of physical qubits, and it remains to be seen whether SQC’s strategy of prioritizing qubit quality can circumvent this requirement at scale.
The Future: High-Fidelity Scaling or a New Bottleneck?
SQC’s demonstration reinforces the idea that error rates, not just qubit count, are a central obstacle in quantum computing. The results suggest that focusing on high-fidelity qubits could reduce the need for massive error correction overhead—but only if this approach can be scaled.
Ultimately, the significance of this work will depend on what comes next. If SQC can maintain these fidelity levels as they scale beyond four qubits, it could provide a more efficient path toward fault-tolerant quantum computing than architectures relying on brute-force qubit increases. However, if scaling introduces new sources of noise or reduces fidelity, then error correction-heavy approaches may still dominate the field.
By demonstrating Grover’s algorithm without error correction, SQC has made a notable step forward in proving the viability of high-fidelity quantum hardware. But the real test will come when these results are extended beyond four qubits—where theory meets the full complexity of quantum reality.
Contributing authors on the paper include I. Thorvaldson, D. Poulos, C. M. Moehle, S. H. Misha, H. Edlbauer, J. Reiner, H. Geng, B. Voisin, M. T. Jones, M. B. Donnelly, L. F. Peña, C. D. Hill, C. R. Myers, J. G. Keizer, Y. Chung, S. K. Gorman, L. Kranz, and M. Y. Simmons