Insider Brief
- Researchers have demonstrated that relativistic quantum fields can act as “universal embezzlers,” allowing quantum states to be extracted with minimal disturbance, thanks to their infinite entanglement properties.
- The study highlights the unique classification of quantum fields through von Neumann algebras, suggesting potential pathways to identify more practical systems with similar entanglement capabilities.
- While theoretically groundbreaking, the findings face challenges in real-world applications, particularly in gravitational contexts where universal embezzlement may not be feasible.
Quantum embezzlement might sound like the inciting element in a Philip K. Dick novel that you tell everyone you’ve read, but actually haven’t; however, researchers report in a new study that quantum embezzlement describes actual quantum states that can be extracted with minimal disturbance.
The study, published in an earlier version on arXiv and in press in Physical Review Letters, connects this counterintuitive phenomenon to the mathematics underlying relativistic quantum fields, suggesting these fields are universal resources for quantum entanglement.
In the words of the researchers, this creates, “an operational characterization of the infinite amount of entanglement present in the vacuum state of relativistic quantum field theories.”

The researchers investigated the process of “embezzling” quantum entanglement — a method of extracting entangled states from an auxiliary quantum system, called an embezzler, without significantly altering its state.
No Entanglement Bank Robbers Just Yet
This discovery provides a practical interpretation of the infinite entanglement present in the vacuum states of such fields. While the main thrust of the work is to prove that it is possible, it does not necessarily create a plan to tap this infinite reservoir for practical purposes, John Cardy at the University of Oxford, told New Scientist.
Quantum entanglement, which is considered the backbone of quantum technologies, has been created and studied through methods more accessible than the complex manipulation of relativistic quantum fields. As noted in New Scientist, contemporary researchers have also actually developed practical techniques to generate entanglement that do not rely on the theoretical constructs explored in this study.
However, this doesn’t mean that the study is merely a theoretical curiosity, according to the researchers, as reported by New Scientist.
One of the research team members, Alexander Stottmeister, of Leibniz University Hannover, explained to New Scientist that relativistic quantum fields possess a kind of infinity unmatched by any experimentally accessible quantum objects. However, the study’s insights into how these fields enable entanglement embezzlement could guide researchers toward identifying more practical quantum systems with similar, though not identical, properties. These systems might not be as ideal for embezzling but could still serve as effective resources for entanglement-based tasks.
Thee study also offers a framework for classifying infinite quantum objects based on their entanglement properties, said another co-author, Henrik Wilming, as reported by New Scientist. The team’s calculations revealed that different fields contain distinct types of entanglement, which can be ranked according to how easily they support embezzlement. This classification may provide a deeper understanding of the operational significance of entanglement across various quantum systems.
Methods and Analysis
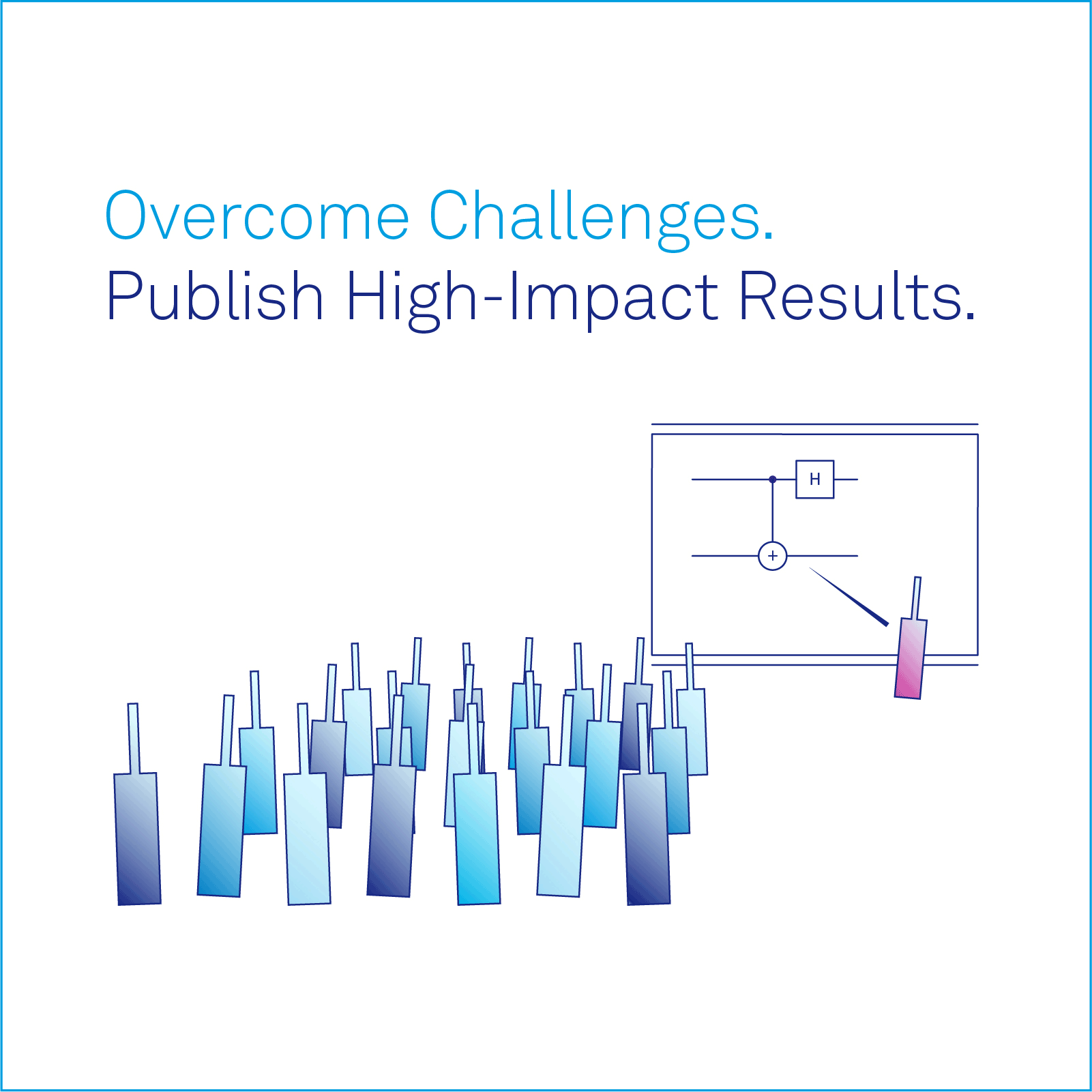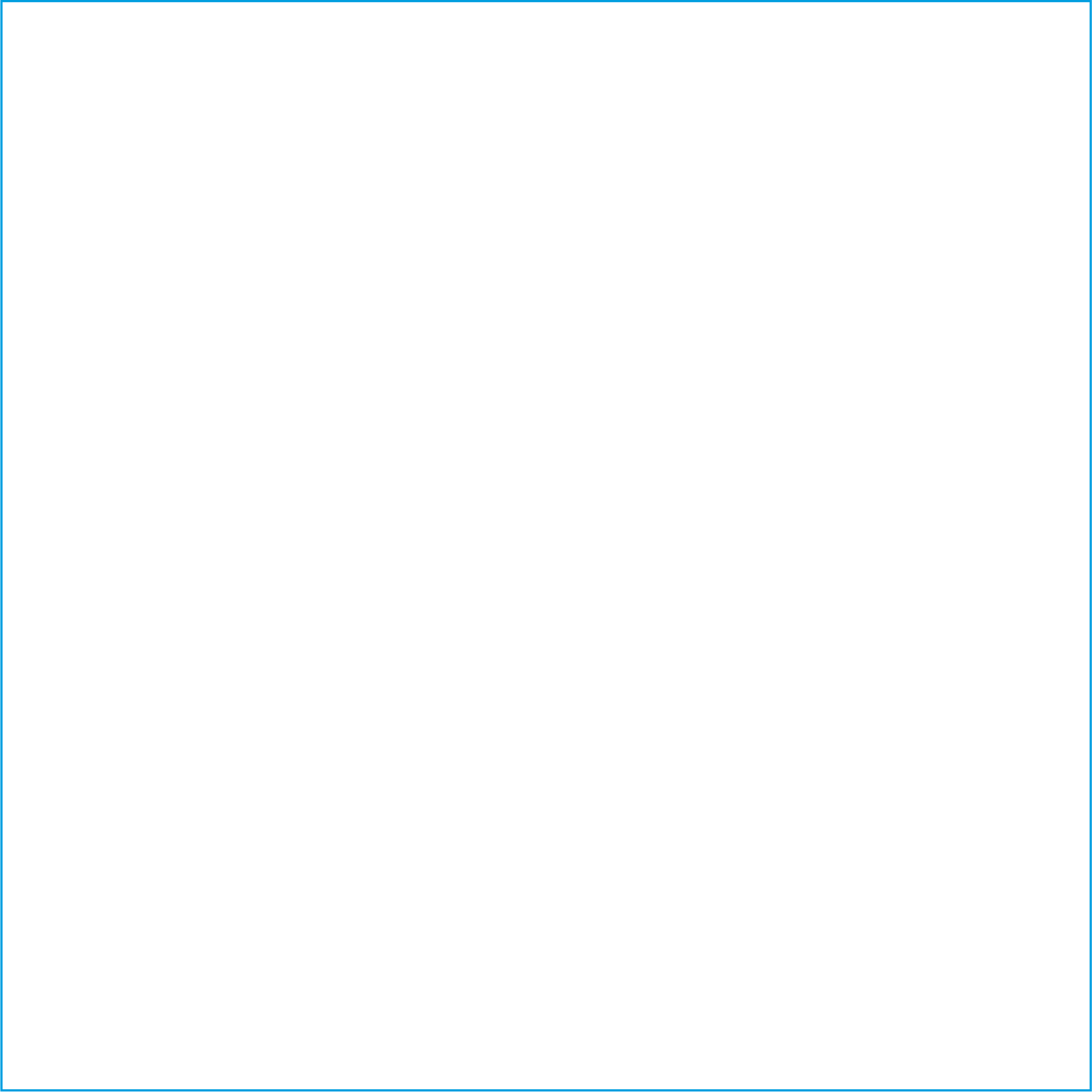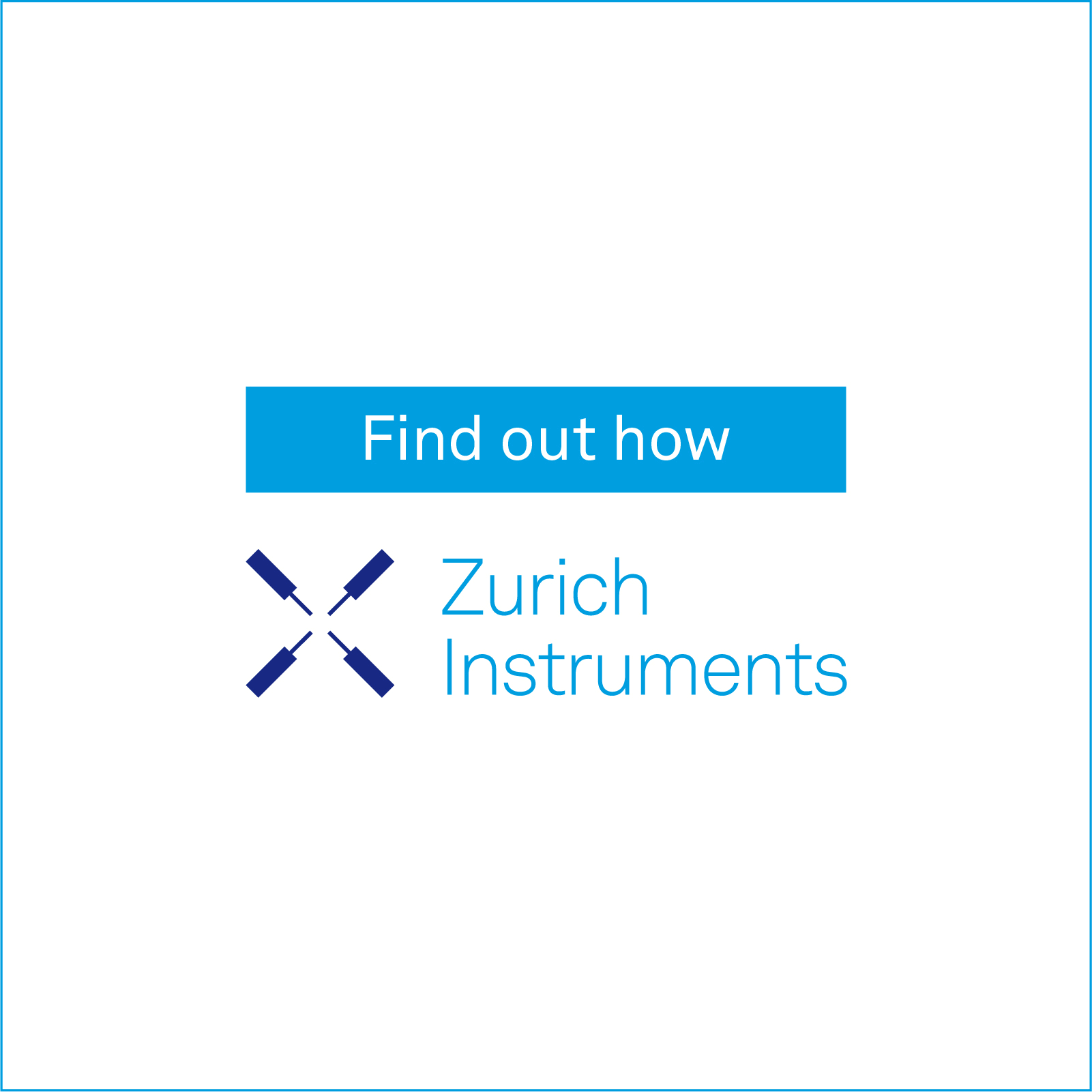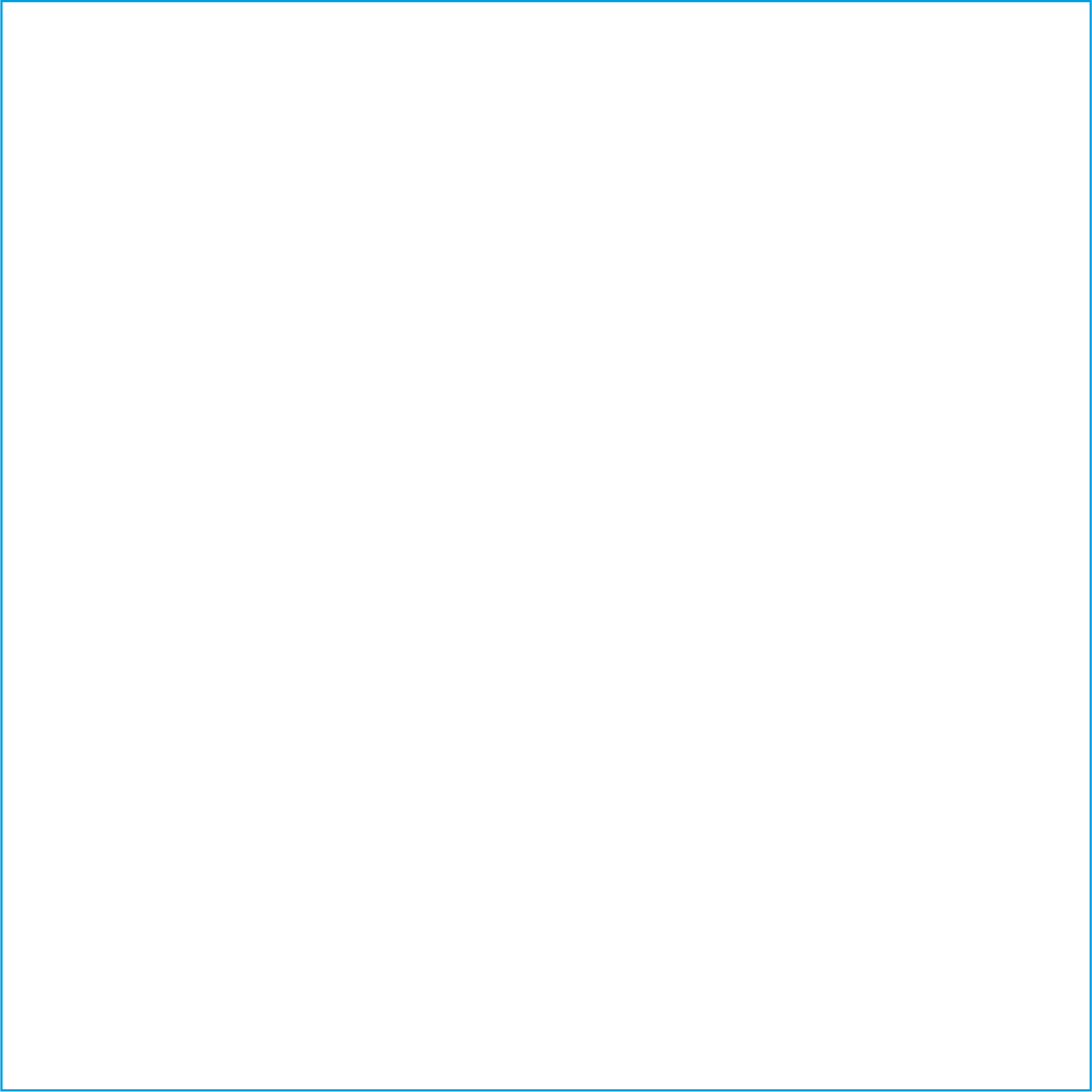
The research hinges on the mathematical classification of von Neumann algebras, abstract structures used to describe quantum systems. These algebras are divided into types, with Type III algebras, specifically Type III1_11, showing unique properties that allow for universal embezzlement. According to the study, relativistic quantum fields fall into this category, meaning they can provide entangled states of any size and precision without fundamentally altering their structure.
The team employed advanced mathematical tools, including modular theory and the classification of quantum states, to establish this connection. They also built on prior work by van Dam and Hayden, who demonstrated that certain quantum states could support embezzlement under specific conditions.
In scientific terms, in quantum mechanics, entangled particles share correlations stronger than anything classical physics can explain. These correlations are vital for quantum computing and secure communication protocols like quantum key distribution.
Typically, using entanglement depletes this resource. Embezzlement, however, defies this logic. By leveraging specific properties of quantum systems, it allows new entangled states to be created while barely diminishing the original resource.
This probably sounds very abstract. (God knows it feels that way to write it.) But, to grasp quantum embezzlement, it might help to focus in on that “entanglement as a resource” concept, a resource, like, to keep with the analogy, money. We can think of entanglement as a type of currency in the quantum world — a valuable resource that powers essential processes like quantum computing and secure communication. Like money, using entanglement typically means spending it, leaving less for future tasks. Quantum embezzlement, however, is like withdrawing funds from a shared account without anyone noticing the balance has changed, enabling the extraction of value while leaving the original resource seemingly untouched.
“Since the bank is in the same state before and after the embezzlement, that means that no one can detect it. It’s the perfect crime,” Lauritz van Luijk tells New Scientist.
The study also points out that relativistic quantum fields, which describe phenomena like the vacuum of space, are uniquely suited to this task.
Limitations and Open Questions
Despite these insights, the study does offer limitations. The theoretical framework assumes idealized conditions, such as perfect control over the quantum system and infinite precision. Real-world applications, particularly in gravitational settings, could disrupt these assumptions. For instance, the study cites previous work suggesting that gravitational effects might change the type of von Neumann algebras associated with quantum fields, potentially eliminating universal embezzlement altogether.
While the study demonstrates the feasibility of embezzlement in principle, practical implementation remains elusive. The researchers note that achieving high precision requires manipulating increasingly large regions of space, which could be challenging in experimental settings.
Future Directions
The findings — and the limitations listed above — point to several avenues for future research. One pressing question is whether the infinite entanglement observed in quantum fields can be harnessed for practical applications. The study hints at the possibility of using localized regions of spacetime to access this resource, but detailed protocols remain to be developed.
Another intriguing area is the potential impact of gravity. If gravitational systems indeed lack universal embezzlement capabilities, this could serve as a distinguishing feature between conventional quantum field theories and theories of quantum gravity. Understanding these differences might provide new insights into the unification of quantum mechanics and general relativity.
The study also invites exploration into multipartite embezzlement, where entanglement involves more than two parties. Initial results suggest that relativistic quantum fields could support such configurations, but the practical and theoretical challenges are significant.
Reinhard F. Werner, also od Leibniz Universität Hannover, was a member of the research team.
For a deeper, more technical look at the study, please review the papers on arXiv and in press in Physical Review Letters.