Written by Mumtaz Gababa, Karthik Narayanan, Elias Lehman, and Alexa Ramirez
In Partnership with Quantum Computing at Berkeley
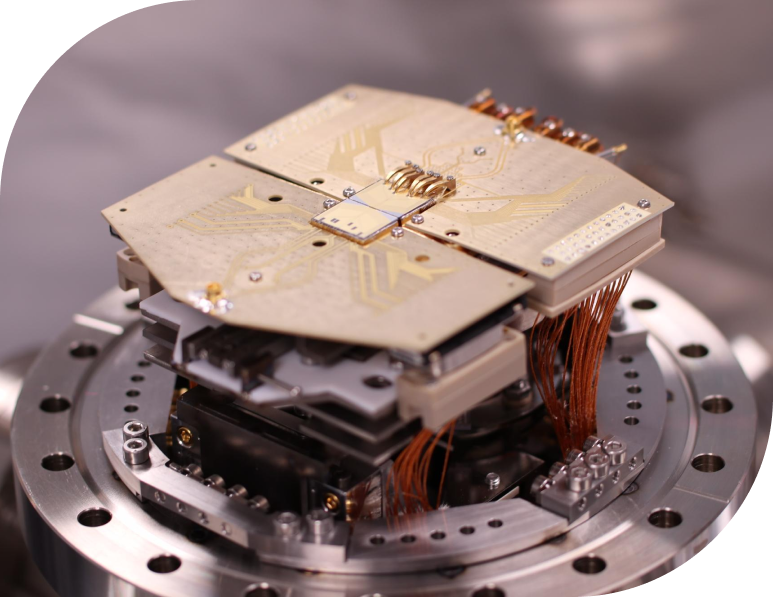
The above image was kindly provided by Universal Quantum

SECTION 1
To build a gate-based quantum computer, a set of criteria must be achieved. DiVincenzo’s criteria, proposed by theoretical physicist David P. DiVincenzo, explains the physical implementation requirements for quantum computers, which include a set of conditions that have to be met to achieve a practical computation. The criteria are the following:
To meet these criteria, a quantum computing architecture must be able to manipulate and control individual qubits, perform operations on them using universal gates, correct errors that may occur during computation, and be able to scale up to large numbers of qubits by suitably connecting them. Meeting these criteria is the primary challenge for researchers, forcing them to develop novel approaches that can achieve these requirements.

SECTION 2
There are several methods for building a quantum computer, each with its advantages and challenges. A common feature of these methods is that they are all designed around the use of qubits. The most suitable approach to building a quantum computer depends on the specific goals of the manufacturer, which determines what form of qubits to use, and thus the overall structure.
Several leading quantum computer manufacturers use trapped ions. These are charged atoms that are held in place by an electric or magnetic field and can be controlled using lasers. This method provides a high level of control over the qubits. The advantage of using trapped ions is that they can remain coherent, or in a state of superposition, for long periods of time. Long coherence times are required to leverage the quantum mechanics of the qubits, perform many operations, and take accurate measurements. However, trapped ions are sensitive to noise, making them prone to decoherence if they interact with the outside world. As a result, much of the research to develop trapped ion quantum computers is in isolating the qubits.
Another leading manufacturer and electronic giant IBM uses superconducting qubits, which are based on circuits that can conduct electricity with zero resistance. These qubits are easy to scale but are sensitive to noise and can be difficult to control, particularly without cryogenic cooling. One advantage of superconducting qubits is that they can achieve quick gate operations–so long as the operation preserves the information with high fidelity.
That is, the gate operations must produce the intended result. This is a leading sector of development in this subfield of quantum computing. Unfortunately, superconducting qubits have relatively short lifetimes compared to other types of qubits.
Semiconductor materials such as enriched silicon and germanium are well-studied materials that are also being developed into qubits by tech conglomerates such as Intel. These qubits can be controlled using electric and magnetic fields, and have the advantage of being relatively easy to fabricate using existing semiconductor technology. They also operate at higher temperatures than superconducting qubits. However, they are prone to decoherence from external fields.
There are other methods for designing qubits, including using the quantum properties of polarized light, the controllability of neurally charged atoms, and the fascinating study of topology.
Ongoing research is focused on reducing noise and improving error rates, to develop efficient, fault-tolerant quantum computers just over the horizon.
The choice of which method to use depends on how the trade-offs between the various characteristics of each method interact with the application the computer is designed for, ie. the manufacturer’s goals. Ultimately, the success of a quantum computer depends on its ability to maintain high gate fidelity and connectivity between qubits, especially while scaling.
SECTION 3
The prototypical qubit is based on the electron spin. When an electron is placed in a magnetic field, the well-known Zeeman effect splits the quantum mechanical spin state into two, known as spin up and spin down. These states can be used as the basis for defining qubit states. Under certain conditions, by applying specific magnetic fields, we can place an electron in a superposition of the up and down states.

One way to isolate and control electrons with the precision needed for quantum computing is to use solid-state systems. In a semiconductor, for example, the energy states of the electrons are organized into bands separated by gaps. The conduction band is the high-energy band, above the gap, and the valence band is the low-energy band, below the gap. Electrons in the conduction band are charge carriers–conductive materials have many of these, while insulators have very few. We say that semiconductors are “doped” with impurities to alter their electrical properties, and the resulting p-type and n-type semiconductors can be used to create devices such as transistors. These principles are used to create quantum dot systems, in which electrons are confined in a small region on the lattice of the solid-state semiconductor, and their spin states can be controlled and manipulated for use as qubits.
Electron spin quantum computers are a promising method, especially because of their scalability using today’s semiconductor manufacturing technology. By using a semiconducting material such as gallium arsenide, electrons can be isolated and controlled as needed to make a qubit.
However, gallium arsenide has an abundance of nuclear spins
which can interact with the electron spin qubit and result in an unknown phase. To perform high-fidelity qubit operations, it is important to maintain precise control over the phase of the qubit, as any lost information will result in decoherence. To reduce this effect, materials such as silicon and germanium are being studied, as they contain isotopes with an even amount of nuclear spins, such that there is no net spin interaction. Silicon and germanium can also be purified by industry, using the same techniques applied to make wafers for electronic applications.
Purified silicon and germanium can be used to build qubits which are then operated using a magnetic field of order one Tesla. This magnetic field results in an energy of micro electron volt, which corresponds to a frequency of GHz. This frequency is the Larmor frequency and is used to keep track of the phase of the qubit. Again, keeping track of the phase, preventing the loss of information, is important for executing quantum algorithms and is done by controlling the parameters that determine the interaction between the electron and the lattice, such as separation between atoms. By controlling these parameters, the properties of the electron can be engineered to build optimal qubit parameters.
SECTION 4
An LC oscillator is an electrical circuit containing an inductor and capacitor in series. When brought to low enough temperatures such that the wires begin to conduct electricity without dissipating energy, better known as superconductivity, this circuit exhibits quantized energy levels. Introducing a unique component called the Josephson junction spaces the levels unevenly, giving the circuit a quality is called anharmonicity which is critical for creating controllable energy levels in any qubit. This device composed of a capacitor and Josephson junction at superconducting (extremely low) temperatures is the general scheme of a superconducting qubit. By customizing the properties of each component in the circuit, researchers are able to create energy levels and spacing however they please, like an artificial atom, in contrast to being restricted to the inherent energy levels of a natural atom. Major players in the classical computing industry such as IBM and Google, in addition to growing names in quantum like Oxford Quantum Circuits and Rigetti Computing, have adopted this device as their qubit of choice for its variability and relevance to their prior work manufacturing circuits on computer chips.
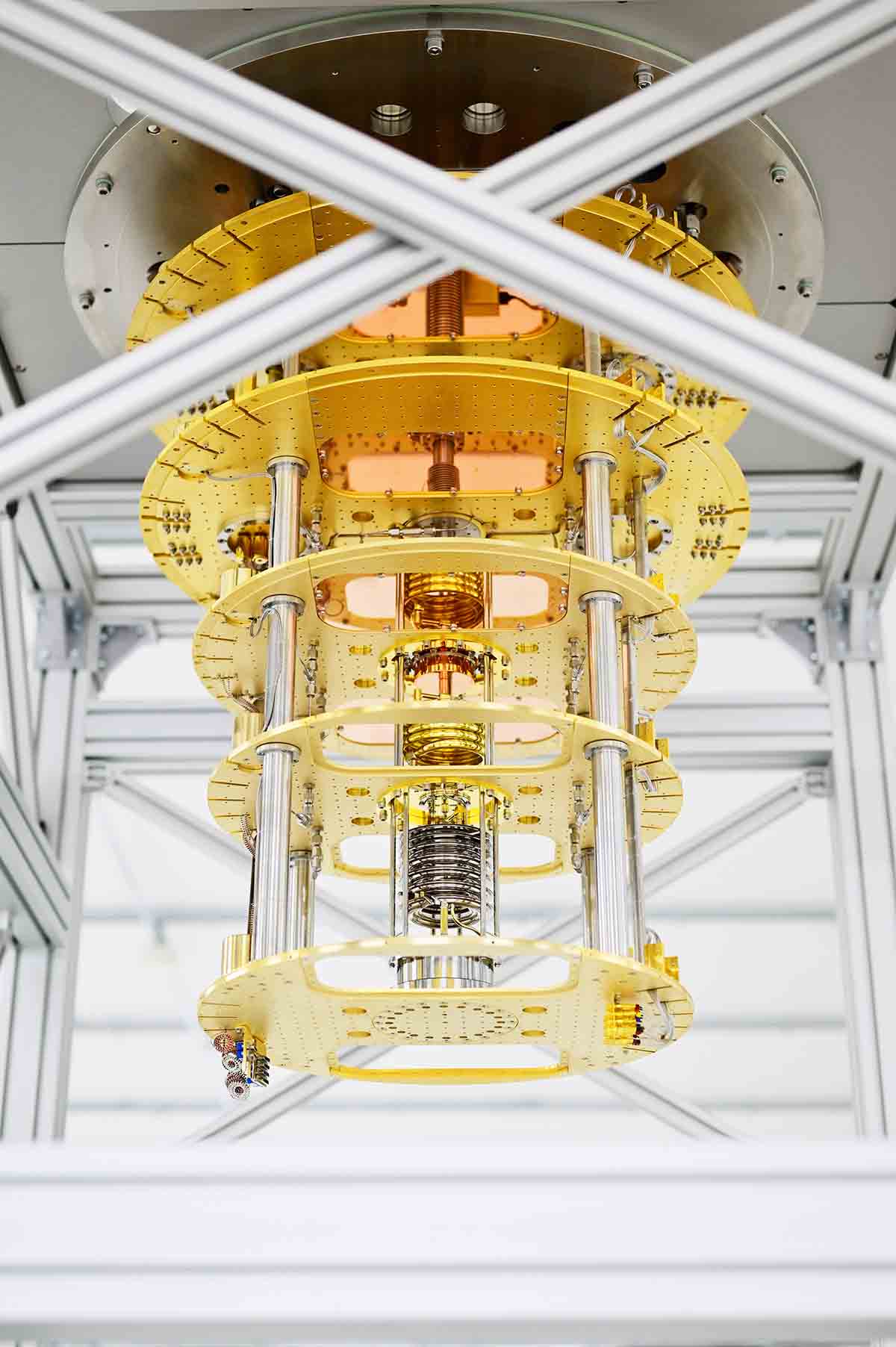
SECTION 5


“The combination of control, stability, scalability, and long coherence time makes the trapped ion model a promising platform for building large-scale quantum computers.”
SECTION 6


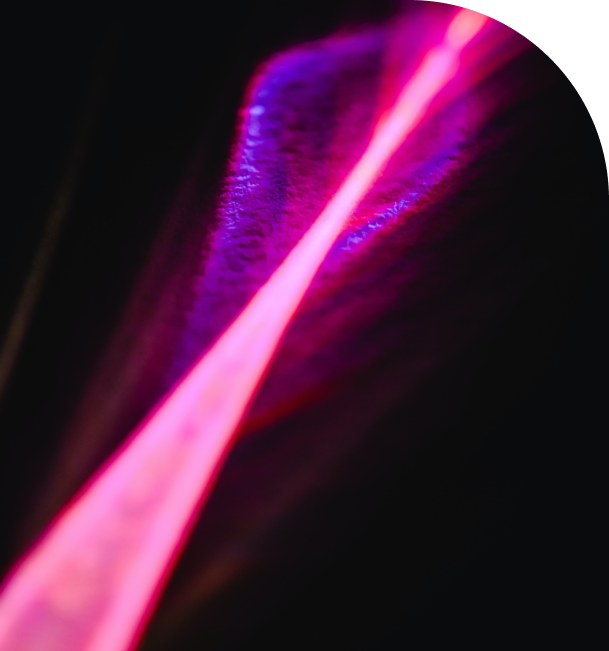
Photons, or particles of light, exhibit quantum behaviors of their own allowing them to be utilized for qubits. Unlike trapped ions and neutral atoms that require cryogenic or laser cooling, photonic qubits can operate at room temperature, making them a promising candidate for building large-scale quantum computers. A leading manufacturer of photonic quantum computers, Psi Quantum, refrigerates the systems to about 4 Kelvin using liquid Helium to optimize the fault tolerance of its photodetectors, whereas competitor Xanadu does not cool the qubits, just the photodetectors to less than 100mK.
The main method of building photonic quantum computers is the KLM protocol developed in 2000 where a light source that produces as few coherent photons as possible travels through fiber optic cables where the qubit states are based on the photon’s modes of polarization. In general, photons can exhibit infinite modes of polarization; however, in the KLM protocol, the photons are either in vertical or horizontal modes as the probability of a photon occupying more than one mode is zero. Therefore, a qubit state can be represented by a photon being in either of these two modes where these states are known as Fock states.
To implement qubits and gates, linear optical elements such as mirrors, beam splitters, and phase shifters are used. Beam splitters can split a beam of photons into two or more separate beams, leading to superposition, and phase shifters can change the phase of the photons which becomes important when entangling them. The combinations of beam splitters, mirrors, and phase shifters lead to a wide variety in the creation of multi-qubit quantum gates. Creating entanglement is more difficult as photons do not interact with each other; however, through a series of phase shifters, photons traveling through different fiber optic cables, entangled states can be created where many of the states that do not become entangled are filtered out.
To determine these final states, photodetectors are used where in a given elementary quantum gate, the photon in the top mode channel corresponds to a state of 0, while a photon in the bottom channel corresponds to a state of 1. As the combination of beam splitters, mirrors, and phase shifters can lead to non-deterministic gates, measuring the fidelity or accuracy of these gates can be very difficult as the operations would have to be performed repeatedly and can become exponentially larger as the number of gates increases. To circumvent this, researchers have used a phenomenon known as quantum teleportation to decrease the time and required resources to determine the fidelity of these systems. Instead of doing these operations successively, each gate would be operated offline and the event signal would be sent back to the quantum circuit using quantum teleportation.
By using photons, these quantum computers can operate at room temperature and through a combination of a few linear optical tools, a universal set of quantum gates can be created. Although using photons as the source of qubits is a seemingly robust system, photons can escape to their environment like any system. However, this is a problem as photons are in a superposition of Fock states, photons escaping can change the state of the photons within the circuit. As phase shifters are used to change the phase of photons, phase decoherence can occur where the phase of the state can change due to factors such as including noise in the control signals that are used to manipulate the quantum system, imperfections in the physical components of the system, and other sources of noise and perturbation. This can become problematic, especially when it can lead to unentangled pairs or affect successive quantum gates in a circuit.
Regarding DiVincenzo’s Criteria, the photonic quantum computer again does theoretically fulfill all five criteria; yet, photon loss, phase decoherence, and efficiency of the quantum gates remain an issue. Simpler methods of photonic quantum computers are being developed to address these issues, hopefully leading to a scalable, fully functional quantum computer.
SECTION 7
Another method of building a quantum computer is using neutral atoms or atoms that have no net electrical charge. Although ions are easy to trap due to their charge, neutral atom quantum computers are less sensitive to stray electric fields, making them a promising candidate for quantum processors. Not just any neutral atom can be used to create these quantum processors, but group 1 or 2 alkali metals such as rubidium are mainly used as its one valence electron gives an electronic structure similar to that of hydrogen.
The method of trapping neutral atoms is not the same as ions since they do not respond to electric fields as well due to their neutral charge; however, they can be trapped by light using a method such as optical tweezing (magneto-optical trap). It uses the optical gradient force of a highly focused laser beam to trap and manipulate small particles or in this case neutral atoms. The particles are cooled to very low temperatures and are trapped in the focus of the laser beam, which creates a strong attractive force that suspends the particles in place in an ultrahigh vacuum. Then, a second laser passes through aperture lenses, creating a strongly focused beam that can act as a “tweezer” where it can control the path and motion of these neutral atoms.
From the optical tweezing method, creating qubits is very similar to the trapped ion case where two electronic levels of a neutral atom can be manipulated and controlled using lasers to create an optical qubit. As there are infinite discrete energy levels in an atom, there can be many configurations of the two electronic states used. Some alkali metals present with a hyperfine structure as rubidium where these hyperfine states are separated by microwave frequencies. By creating hyperfine qubits, the small energy gap between hyperfine states allows for an electron to stay in an excited state for a very long time before decoherence occurs. Therefore, hyperfine qubits have longer coherence times than their optical counterparts. However, these qubits are still susceptible to decoherence as their unpaired electron can interact with unwanted magnetic fields or unfavorably couple to the tweezer light.
To scale up to a two-qubit system or more, the qubits are stored in an array of optical traps, which are created by an incident beam being split up into many beams that can be focused through aperture/objective lenses. Because neutral atoms can only interact with one another through contact collisions, the only way these atoms can interact is by dipole forces if they present with a large electric dipole moment.



To create these sizeable electric dipole moments between two atoms, one of the atoms is targeted by a laser pulse that can drive the atom into a highly excited electronic state known as a Rydberg state. The Rydberg state causes a phenomenon known as a Rydberg blockade where a strong electric dipole moment is created, preventing the other atom from excitation. As such, these two atoms are entangled, a key feature needed for the implementation of quantum algorithms and protocols.
To measure the qubits, fluorescence images can be collected (through an electron-multiplying charge-coupled device) where qubit state 0 will appear as bright while 1 will appear as dark. Other methods to measure qubits include state-selecting imaging or electron shelving by resonant absorption imaging or electromagnetically induced transparency.
With the neutral atom method, some computers have scaled to over one hundred qubits. Unlike trapped ion qubits, these neutral atoms weakly interact with their environment, becoming less susceptible to stray electric or magnetic fields. Therefore, scaling neutral atom quantum computers is primarily limited by the system that generates the magneto-optical trap. Yet, scalability remains an issue due to the large experimental setup required to trap these atoms.
The method of using optical tweezers is a powerful tool in trapping neutral atoms, but there are factors such as trapping efficiency and spatiotemporal resolution that limits its ability to trap these atoms effectively. Optical tweezers have a low trapping efficiency, which means that not all particles that are exposed to the laser beam will be trapped. This can be particularly challenging when trying to trap large numbers of particles. The spatial resolution of optical tweezers is limited by the diffraction limit, which means that the position of the trapped particle can only be determined with a certain level of uncertainty. This can limit the precision of measurements and manipulations that are performed using optical tweezers. The temporal resolution of optical tweezers is limited by the laser pulse duration and other factors, which can limit the speed at which measurements and manipulations can be performed.
Regarding DiVincenzo’s Criteria, the neutral atom again does theoretically fulfill all five criteria; yet, scalability remains an issue due to the complicated setup to trap these atoms.
Overall, neutral atom quantum computers are a promising approach to quantum computing and have the potential to enable the development of powerful quantum algorithms and protocols.
SECTION 8

Models of topological QC rely on a quasi-particle called an anyon. Crucially, these particles live on a 2-dimensional surface and are an emergent property of the underlying physics of a system. Consider 2 anyons, anyon A and anyon B, restricted to move on a 2D surface (like a sheet of paper). Keep anyon A still, and move anyon B in a loop around anyon A, bringing it back to its original position. Although we are back where we started, in 2D this path is topologically different from doing nothing at all, since we cannot mold a path that does go around anyon A into a path that does not, without hitting against anyon A. In 3D, we can simply take the loop under anyon A, and mold this path into a dot without hitting against anything, as we have one more dimension to work with.

There are several challenges involved in the physical realization of topological QC models. Chief amongst them is verifying whether systems thought to exhibit anyonic behavior do exhibit the desired properties. Potential candidates include so-called Majorana zero modes in superconducting materials, Majorana zero modes in chiral p-wave Fermi gasses, and vortex-antivortex pairs in Bose–Einstein condensates.
So far, the only system claimed to have been observed is Majorana zero modes in superconductors, in a preprint by Microsoft researchers in July 2022. Majorana zero modes have yet to be robustly proven viable for topological quantum computing.
Topology is a field of mathematics that studies properties of geometrical objects that are invariant under continuous transformations. Think of molding a piece of clay, without tearing it, creating holes in it, or sticking it to another piece. To a topologist, a doughnut is the same as a coffee cup: both have the same number of holes and can be continuously (in the above sense) molded from one to another. The idea is this: if the information on a quantum computer were encoded in a material’s topological properties, then small disturbances to the system (molding the clay), will not destroy this information. It would be topologically protected!

So for a 2D physical system where topology matters, simply taking one particle in a loop around another has the potential to induce a physical change to the system, as it is topologically different from doing nothing. This is the essence of topological QC. Models of such anyonic systems include Fibonacci anyons and Ising anyons. In these models, looping particles around each other (called ‘braiding’) induces unitary transformations, which can be combined to form quantum gates such as NOT, CNOT, and the Hadamard gate. Information is topologically protected because it is manipulated and stored in the bulk of the system and not in any one particle.

Currently, systems are not being commercially engineered using this approach.
The difficulty in verifying anyonic behavior, as evidenced by Microsoft’s earlier struggles with Majorana zero modes is far outweighed by the improvements in decoherence times and inherently lower error rate due to topological QC, which has a real chance of being the preferred method of quantum computing soon.
SECTION 9
Unlike other deep technology industries, which often advance primarily in national labs and universities, the frontier of quantum computing is being pushed in the private sector. The only computers available to the public are commercially produced and they are quickly evolving. The Quantum Insider’s intelligence platform features a qubit roadmap to best understand the trajectories in which this industry is heading.
One of our team will be in touch to learn more about your requirements, and provide pricing and access options.